Deflection and Delay of Light

Everybody knows that light travels in straight lines, but while that is its natural tendency light can be deflected by lenses, mirrors, and by gravitational fields. Newtonian mechanics predicts that a particle traveling at the speed of light which just grazes the edge of the Sun will be deflected by 0.875 seconds of arc. That means that the image we see of a star will be displaced away from the Sun by this angle. The figure below shows this with the black showing the situation when the Sun is not close to the star. When the Sun is nearly blocking the star its image is deflected outward giving the red image. This Newtonian model also predicts that the gravitational attraction of the Sun will make light travel faster close to the Sun, so according to Newton the deflected light arrives before the undeflected light. The figure shows the red light pulse arriving before the black light pulse. Of course the travel time for starlight is very hard to measure, and the deflection of starlight can only be measured during a total eclipse of the Sun. The deflection angle is actually very small, and in the figure it has been increased by a factor of nearly 10,000 for clarity.

Before Einstein developed the full theory of General Relativity he also predicted a deflection of 0.875 arcseconds in 1913, and asked astronomers to look for it. But World War I intervened, and during the war Einstein changed his prediction to 1.75 arcseconds, which is twice the Newtonian deflection. The final Einstein prediction is shown in green in the figure above. An expedition to a solar eclipse in 1919 measured this larger value for the deflection. Currently the deflection of "light" is best measured using radio astronomy, since radio waves can be measured during the day without waiting for an eclipse of the Sun. Lebach et al. (1995, PRL, 75, 1439) find a deflection of 0.9998 +/- 0.0008 times Einstein's prediction. This agrees with Einstein within 0.3 standard deviations, but differs from the Newtonian deflection by 600 standard deviations.

Einstein predicts that light will be delayed instead of accelerated when passing close to the Sun. Notice in the figure above that the green light pulse arrives after the black light pulse. This effect is closely related to the deflection of starlight. Since times can be measured to much greater accuracy than arcsecond angles, the greatest accuracy on this effect is now given by measuring the time delay instead of the angle. In order to measure the time delay one needs a a spacecraft behind the Sun instead of a star. This was first done by Irwin Shapiro (Shapiro et al. 1977, JGR, 82, 4329), and most recently done by Bertotti, Iess & Tortora (2003, Nature, 425, 374-376). The current result is 1.00001 +/- 0.000012 times the general relativity prediction, or -2.000021 +/- 0.000023 times the Newtonian prediction. So the delay observations agree with Einstein within 0.9 standard deviations, but are 130,000 standard deviations away from the Newtonian prediction.

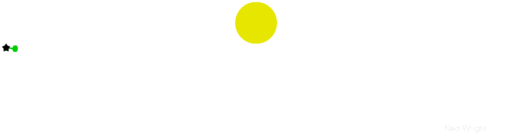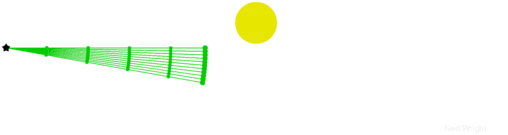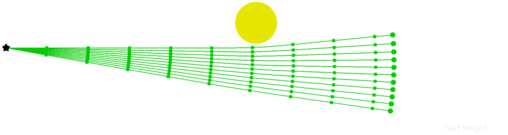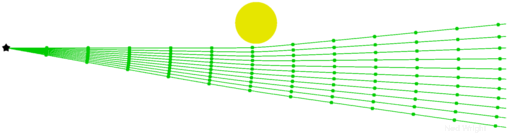
In a very real sense, the delay experienced by light passing a massive object is responsible for the deflection of the light. The figure below shows a bundle of rays passing the Sun at various distances. The rays are always perpendicular to the wavefronts which mark the set of points with constant travel time from the star. In order to bend the light toward the star one needs to delay the wavefront near the star.

Another way to delay the wavefronts of light is to send the light through glass, as in a lens or a prism. The deflection and delay of light caused by massive objects is called gravitational lensing.